|
Schedule of the conference with abstracts
2009/11/05,
Thursday morning session
|
Time:
9:10-10:00
Speaker:
Eiji Yanagida
Affiliation:
Tohoku University,
JAPAN
Email: yanagida.AT.math.tohoku.ac.jp
Title: Connecting orbits for a
semilinear parabolic equation with a supercritical exponent
Abstract:
|
|
Time:
10:10-10:50
Speaker: Sun-Sig Byun
Affiliation: Seoul National University
Email: byun.AT.snu.ac.kr
Title: Gradient Estimates for Elliptic and
Parabolic PDEs with Measurable Coefficients in Nonsmooth Domains
Abstract: We find a minimal
regularity requirement on the coefficients and a lowest level of geometric
condition on the boundary for a classical Calderon-Zygmund estimate.
|
|
Time:
11:00-11:40
Speaker: Minkyu Kwak
Affiliation: Chonnam National
University
Email: mkkwak.AT.jnu.ac.kr
Title: The cone property for parabolic
partial differential equations
Abstract: We show that a class of parabolic partial
differential equations satisfy a cone property. This result implies that
the projection from the global attractor to a finite dimensional Fourier
space is injective and thus the global attractor becomes a finite dimensional
graph.
|
|
Time:
11:50-12:30
Speaker: Ki-Ahm Lee
Affiliation: Seoul National University
Email: kiahm.AT.math.snu.ac.kr
Title: Parabolic Method for Nonlinear Eigen Value Problems
Abstract: In this talk, we are going
to discuss the parabolic approach on the nonlinear eigen-value problems and
its recent development. The geometric structure of the nonnegative first
eigen function of Linear or Nonlinear eigen value problems has been studied
to understand the ground state in Physics, asymptotic behavior of the
parabolic flows, and its own interest in elliptic PDE. There has been H.J.
Brascamp and E.H. Lieb's method based on probability, and N.J. Korevaar's
method which can be applicable to linear or sub-linear case. Parabolic
approach has been developed to deal with super-linear case. Such idea has
been extended to eigen-value problems for nonlocal equations and fully
nonlinear equations.
|
2009/11/05,
Thursday afternoon session
|
Time:
2:00-2:40
Speaker: Seong-A Shim
Affiliation: Sungshin
Women's University
Email: shims.AT.sungshin.ac.kr
Title: Bifurcation properties of
Holling type predator-prey systems
Abstract: There have been
many experimental and observational evidences which indicate the predator
response to prey density needs not always monotone increasing as in the
classical predator-prey models in population dynamics. Holling type functional response
depicts situations in which
sufficiently large number of the prey species increases their ability to
defend or disguise themselves from the predator. In this paper we
investigated the stability and instability property for a Holling type
predator-prey system of a generalized form. Hopf type bifurcation
properties of the non-diffusive system and the diffusion effects on
instability and bifurcation values are studied.
|
|
Time:
2:50-3:30
Speaker:
Hyundae Lee
Affiliation:
Inha University
Email: hdlee.AT.inha.ac.kr
Title:
Progress on the Strong Eshelby's Conjecture
Abstract: We make progress
towards proving the strong Eshelby's conjecture in three dimensions. We
prove that if for a single nonzero uniform loading the strain inside
inclusion is constant and further the eigenvalues of this train are either all the same or all distinct, then
the inclusion must be of ellipsoidal shape. As a consequence, we show that
for two linearly independent loadings the strains inside the inclusions are
uniform, then the inclusion must be
of ellipsoidal shape. We then use this result
to address a problem of determining the shape of an inclusion when the
elastic moment tensor (elastic polarizability tensor) is extremal. We show
that the shape of inclusions, for which
the lower Hashin-Shtrikman bound either on the bulk part or on the shear
part of the elastic moment tensor is attained,
is an ellipse in two dimensions and an ellipsoid in three dimensions.
|
|
Time:
3:40-4:20
Speaker: Mikyoung Lim
Affiliation: KAIST
Email: mklim.AT.kaist.ac.kr
Title:
Blow-up of Electric Fields between Closely Spaced Spherical Perfect
Conductors
Abstract:
The electric field increases toward
infinity in the narrow region between closely adjacent perfect conductors
as they approach each other. We establish optimal estimates for the
electric field associated with the distance
between two spherical conductors in n-dimensional
spaces for n which is equal to or greater than 2. The novelty of these estimates is that they explicitly
describe the dependency of the blow-up rate on the geometric parameters:
the radii of the conductors.
|
|
Time:
4:30-5:10
Speaker: Jongmin Han
Affiliation:
Hankuk University of Foreign Studies
Email: jmhan.AT.hufs.ac.kr
Title: Existence and asymptotics of
topological solutions in the self-dual
Maxwell-Chern-Simons CP(1) model.
Abstract: In this talk,
we consider the topological vortex equations arising in the self-dual
Maxwell-Chern-Simons $CP(1)$ model. We prove the existence of solutions for
any parameters $\kappa$ and $q$ by variational method. We also verify the Maxwell limit for any multivortex
solutions and the Chern-Simons limit for the variational solutions.
The latter limit hold true for any
solutions when there is only one vortex point and
the parameters satisfy some constraints.
|
|
Time:
5:20-6:00
Speaker: Kwangseok Choe
Affiliation: Inha University
Email: kschoe.AT.inha.ac.kr
Title: Vortex solutions in the self-dual
Chern-Simons-Higgs theory
Abstract: In the Chern-Simons
theories, the Chern-Simons-Higgs model was proposed in an attempt to
explain a certain type of superconductivity. In a static configuration, the
governing equation for the Chern-Simons-Higgs model
is a semilinear elliptic equation with
exponential nonlinearities, and it is
often called the Chern-Simons-Higgs (vortex) equation. We briefly review
recent progress on existence and asymptotic behavior for solutions of the Chern-Simons-Higgs equation.
|
2009/11/06,
Friday morning session
|
Time:
9:00-9:50
Speaker:
Edward Dancer
Affiliation: University of Sydney, AUSTRALIA
Email:
Title:
An unexpected connection between small diffusion problems and problems
with infinite boundary values
Abstract:
|
|
Time:
10:00-10:40
Speaker: Seick Kim
Affiliation:
Yonsei University
Email: kimseick.AT.yonsei.ac.kr
Title:
Global estimates for Green's matrices of second order elliptic systems
Abstract:
|
|
Time:
10:50-11:30
Speaker: Soo Hyun Bae
Affiliation: Hanbat National
University
Email: shbae.AT.hanbat.ac.kr
Title: Singular solutions of semilinear
elliptic equations and the method of phase plane
Abstract:
This
talk expalins how to apply the method of phase plane to classify singular
radial solutions for semilinear elliptic equations with radial coefficient
equipped with proper monotonicity. Particular interest is on the case
involving critical Sobolev exponent.
|
|
Time:
11:40:-12:20
Speaker: Jaeyoung Byeon
Affiliation:
POSTECH
Email: jbyeon.AT.postech.ac.kr
Title:
Bifurcation from the essential spectrum for nonlinear elliptic problems
Abstract:
|
2009/11/06,
Friday afternoon session
|
Time:
2:00-2:50
Speaker:
Chang-Shou Lin
Affiliation: National Taiwan University, TAIWAN
Email: cslin.AT.math.ntu.edu.tw
Title: The minimizers of
Caffarelli-Kohn-Nirenberg inequalities with the singularity on the
boundary of domains
Abstract:

|
|
Time:
3:00-3:40
Speaker: Hyung Ju Hwang
Affiliation: POSTECH
Email: hjhwang.AT.postech.ac.kr
Title: Kinetic model in a chemosensitive
movement
Abstract:
Mathematical models of bacterial
populations are often written as systems of partial differential equations
for the densities of bacteria and concentrations of extracellular (signal)
chemicals. This approach has been employed since the seminal work of Keller and Segel
in 1970s. The system has been shown to permit travelling wave solutions
which correspond to travelling band formation
in bacterial colonies, yet only under specific
criteria, such as a singularity in the chemotactic sensitivity function as
the signal approaches zero. Such a singularity generates infinite
macroscopic velocities which are biologically unrealistic. In this talk, we
discuss a model that takes into consideration relevant details of the
intracellular processes while avoiding the singularity in the chemotactic
sensitivity. We talk about the global existence of solutions and the existence
of travelling wave solutions.
|
|
Time:
3:50-4:30
Speaker: Young-Ran Lee
Affiliation: Sogang University
Email: younglee.AT.sogang.ac.kr
Title: Exponential decay of solutions for
dispersion managed non-linear Schrodinger equation
Abstract: We show that any L2
solution of the dispersion managed non-linear Schrodinger equation with
zero average dispersion decays exponentially in space and frequency
domains. This confirms in the affirmative Lushnikov¡¯s conjecture of
exponential decay of dispersion managed solitons. This is a joint work with
M. Burak Erdogan and Dirk Hundertmark.
|
|
Time:
4:40-5:20
Speaker: Tong Keun Chang
Affiliation:
KIAS
Email: chang7357.AT.kias.re.kr
Title:
Mixed boundary value problem of the Laplace equation
Abstract:
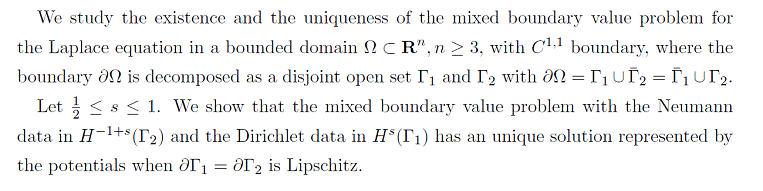
|
|
Time:
5:30-6:10
Speaker: Yonggeun Cho
Affiliation: Chonbuk National
University
Email: changocho.AT.jbnu.ac.kr
Title: Elliptic estimates independent of
domain expansion
Abstract: In this talk, we consider elliptic
estimates for a system with smooth variable coefficients on a domain
$\Omega \subset \mathbb{R}^n, n \ge 2$ containing the origin. By assuming
that the coefficients are in a homogeneous Sobolev space we will see an
invariance of the elliptic estimates under a domain expansion
defined by the scaling $\Omega_R = \{y
: y = Rx, x \in \Omega\}, R> 1$ . These invariant estimates will be
applied to the existence and uniqueness of global strong solution to a
parabolic system on some unbounded domains like whole space, half space or
exterior domain with compact complement.
|
2009/11/07,
Saturday morning session
|
Time:
9:00-9:50
Speaker:
Yanyan Li
Affiliation:
Rutgers University
Email: yyli.AT.math.rutgers.edu
Title:
Some remarks on singular solutions of nonlinear elliptic equations
Abstract:
|
|
Time:
10:00-10:40
Speaker: Jeonhwan Choi
Affiliation:
Korea University
Email: jchoi.AT.korea.ac.kr
Title:
Supercritical gravity waves generated by
a positive force-Theory and Experiment
Abstract:
Two dimensional gravity waves of an idea
fluid are studied when a positive symmetric force is given. We study
KdV theory theoritically and numerically and compare the numerical
results with experimental results.
|
|
Time:
10:50-11:10
Speaker: Yong Jung Kim
Affiliation: KAIST
Email: yongkim.AT.kaist.edu
Title: Asymptotic agreement of
moments and higher order contraction in the Burgers equation
Abstract:
The purpose of this paper is to investigate the relation
between the moments and the asymptotic behavior of solutions to the Burgers
equation. The Burgers equation is a special nonlinear
problem that turns into a linear one
after the Cole-Hopf transformation. Our asymptotic analysis depends on this
transformation. In this paper an asymptotic approximate solution is
constructed, which is given by the inverse Cole-Hopf transformation of a
summation of $n$ heat kernels. The $k$-th order moments of the exact and
the approximate solution are contracting with order
$O\big((\sqrt{t})^{k-2n-1+1/p})$ in $L^p$-norm as $t\to\infty$. This
asymptotics indicates that the convergence order is increased by a
similarity scale whenever the order of controlled moments is increased by
one. The theoretical asymptotic convergence orders are tested numerically.
|
|